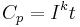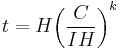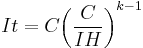Peukert's law

Peukert's law, presented by the German scientist W. Peukert in 1897, expresses the capacity of a lead–acid battery in terms of the rate at which it is discharged. As the rate increases, the battery's available capacity decreases.

Manufacturers rate the capacity of a battery with reference to a discharge time. For example, a battery might be rated at 100 A·h when discharged at a rate that will fully discharge the battery in 20 hours. In this example, the discharge current would be 5 amperes. If the battery is discharged in a shorter time, with a higher current, the delivered capacity is less. Peukert's law describes an exponential relationship between the discharge current (normalized to some base rated current) and delivered capacity (nomalized to the rated capacity), over some specified range of discharge currents. If the exponent constant was one, the delivered capacity would be independent of the current. For a lead–acid battery however, the value of k is typically between 1.1 and 1.3. It generally ranges from 1.05 - 1.15 for VRSLAB AGM batteries, 1.1-1.25 for gel, and 1.2-1.6 for flooded batteries.^[1] The Peukert constant varies according to the age of the battery, generally increasing with age. Application at low discharge rates must take into account the battery self-discharge current. At very high currents, practical batteries will give even less capacity than predicted from a fixed exponent. The equation does not allow for the effect of temperature on battery capacity.

Formula

For a one-ampere discharge rate, Peukert's law is often stated as:

$C_p = I^k t \,$

where:

$C_p \,$ is the capacity at a one-ampere discharge rate, which must be expressed in A·h.

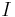$I \,$ is the actual discharge current relative to 1 ampere, which is then dimensionless.

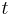$t \,$ is the actual time to discharge the battery, which must be expressed in h.

The capacity at a one-ampere discharge rate is not usually given for practical cells. It is useful to reformulate the law to a known capacity and discharge rate:

$t = H{\left(\frac{C}{I H}\right)^k}\,$

where:

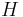$H \,$ is the rated discharge time, in (hours).

$C \,$ is the rated capacity at that discharge rate, in (Ampere-hours).

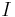$I \,$ is the actual discharge current, in (Amps).

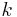$k \,$ is the Peukert constant, (dimensionless).

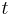$t \,$ is the actual time to discharge the battery, in (hours).

Using the above example, if the battery has a Peukert constant of 1.2 and it is discharged at a rate of 10 amperes, it would be fully discharged in time 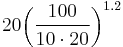$20{\left(\frac{100}{10 \cdot 20}\right)^{1.2}}\,$ which is approximately 8.7 hours. It would therefore dispense only 87 ampere hours rather than 100.

Peukert's law can be written as:

$I t = C{\left(\frac{C}{I H}\right)^{k-1}}\,$

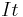giving $I t\,$ which is the effective capacity at the discharge rate $I\,$ .

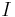Where the capacity is listed for two discharge rates, the Peukert exponent can be determined algebraically.

Peukert's law becomes a key issue in a battery electric vehicle where batteries rated, for example, at a 20 hour discharge time are used at a much shorter discharge time of about 1 hour.

References

W. Peukert, Über die Abhängigkeit der Kapazität von der Entladestromstärke bei Bleiakkumulatoren, Elektrotechnische Zeitschrift 20 (1897)
D. Doerffel, S.A. Sharkh, A critical review of using the Peukert equation for determining the remaining capacity of lead–acid and lithium-ion batteries, Journal of Power Sources, 155 (2006) 395–400

^ http://www.bdbatteries.com/peukert.php Peukert constant vs. battery type

Peukert's law

Formula

References

External links